Excel
5 Ways To Calculate IQR
Introduction to Interquartile Range (IQR)
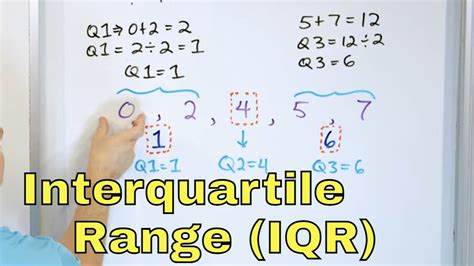
The Interquartile Range (IQR) is a measure of the spread or dispersion of a set of data. It is calculated by finding the difference between the third quartile (Q3) and the first quartile (Q1) of the data. The IQR is a useful statistic for describing the distribution of data and can be used to identify outliers. In this article, we will discuss five ways to calculate the IQR.
Method 1: Using the Quartile Formula
The first method to calculate the IQR is by using the quartile formula. To do this, you need to arrange the data in order from smallest to largest. Then, you find the median (middle value) of the data. The median is also known as the second quartile (Q2). The first quartile (Q1) is the median of the lower half of the data, and the third quartile (Q3) is the median of the upper half of the data. The IQR is then calculated as IQR = Q3 - Q1.
Method 2: Using a Histogram
Another way to calculate the IQR is by using a histogram. A histogram is a graphical representation of the distribution of the data. To calculate the IQR using a histogram, you need to identify the first quartile (Q1) and the third quartile (Q3) on the histogram. The IQR is then calculated as IQR = Q3 - Q1. This method is useful for visualizing the distribution of the data and identifying outliers.
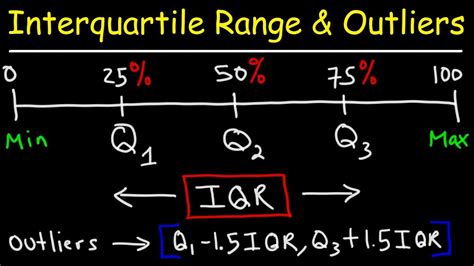
Method 3: Using a Box Plot
A box plot is a graphical representation of the distribution of the data that shows the five-number summary: the minimum, first quartile (Q1), median (Q2), third quartile (Q3), and maximum. To calculate the IQR using a box plot, you need to identify the first quartile (Q1) and the third quartile (Q3) on the box plot. The IQR is then calculated as IQR = Q3 - Q1. This method is useful for comparing the distribution of multiple datasets.
Method 4: Using a Statistical Calculator
If you have a large dataset, it may be more efficient to use a statistical calculator to calculate the IQR. Most statistical calculators have a built-in function to calculate the IQR. Simply enter the data into the calculator and select the IQR function. The calculator will then calculate the IQR for you.
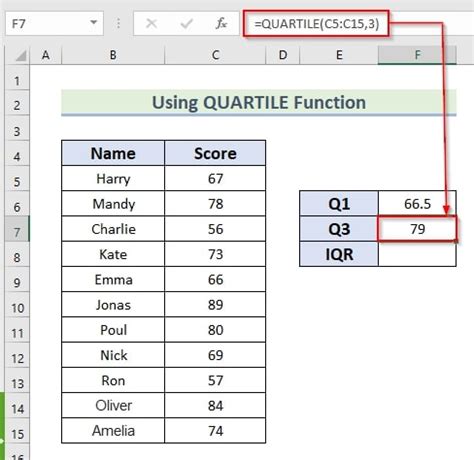
Method 5: Using a Spreadsheet
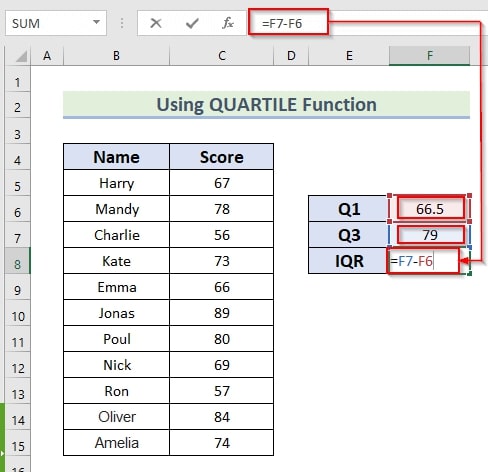
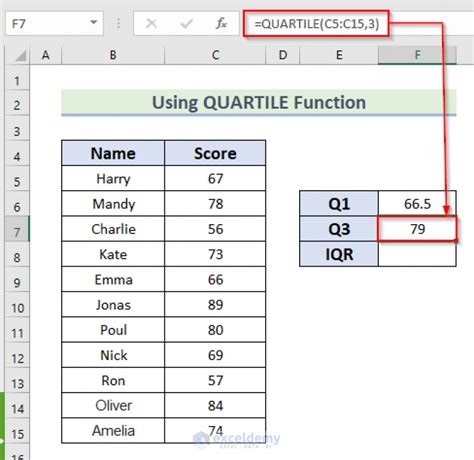
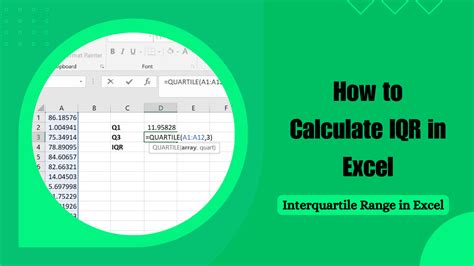
Finally, you can also calculate the IQR using a spreadsheet program such as Microsoft Excel or Google Sheets. To do this, you need to enter the data into a column in the spreadsheet. Then, you can use the QUARTILE function to calculate the first and third quartiles. The IQR is then calculated as IQR = Q3 - Q1. This method is useful for large datasets and can be automated using formulas.
| Method | Description |
|---|---|
| Quartile Formula | Arrange data in order, find median, and calculate Q1 and Q3 |
| Histogram | Identify Q1 and Q3 on the histogram |
| Box Plot | Identify Q1 and Q3 on the box plot |
| Statistical Calculator | Enter data into calculator and select IQR function |
| Spreadsheet | Enter data into spreadsheet and use QUARTILE function |

📝 Note: When calculating the IQR, it is essential to check for outliers in the data, as they can affect the accuracy of the calculation.
To summarize, there are five ways to calculate the IQR: using the quartile formula, a histogram, a box plot, a statistical calculator, or a spreadsheet. Each method has its advantages and disadvantages, and the choice of method depends on the size and complexity of the dataset. By understanding how to calculate the IQR, you can gain insights into the distribution of your data and make informed decisions. The key points to take away are the different methods for calculating the IQR and the importance of checking for outliers in the data.