Calculate Cpk in Excel Easily
Introduction to Cpk Calculation
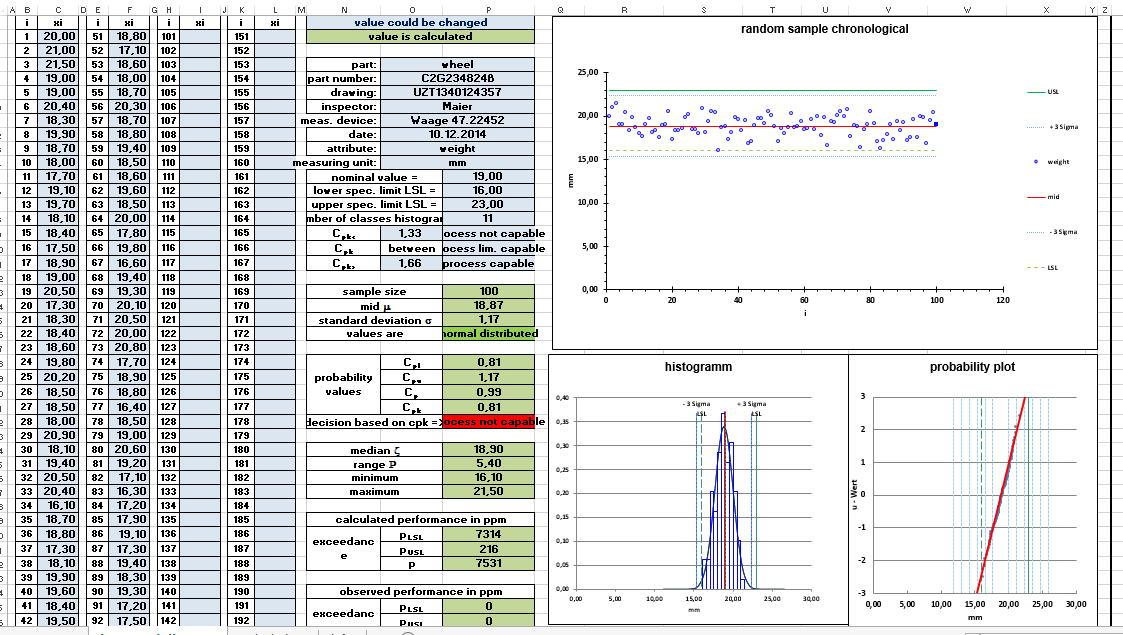
Calculating the Cpk (Capability Index) is a crucial step in understanding the capability of a process to produce output within specified limits. The Cpk is a statistical measure that compares the width of a distribution to the specification limits. A higher Cpk value indicates that the process is more capable of producing output within the desired specifications. In this article, we will explore how to calculate Cpk in Excel easily.
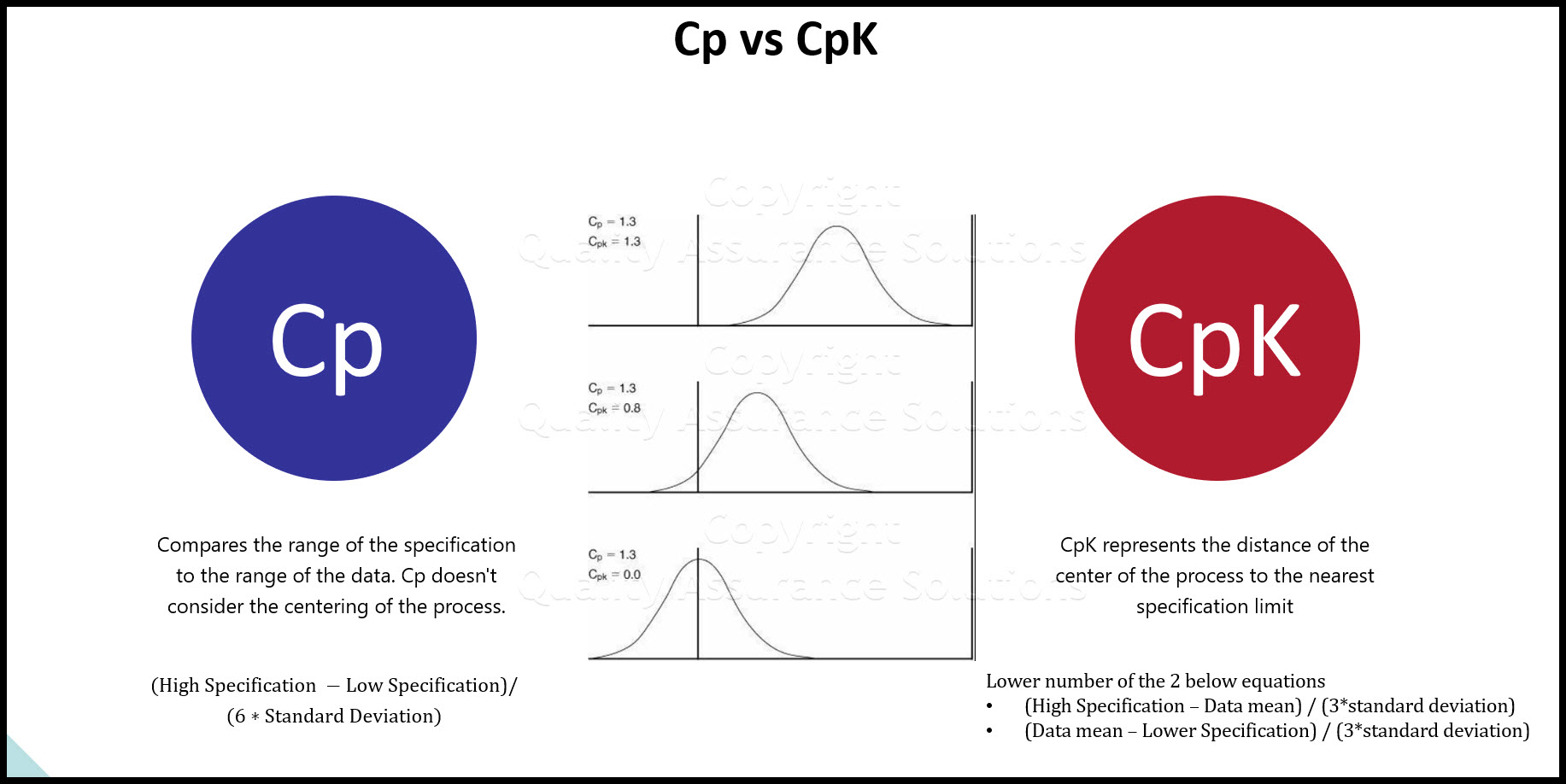
Understanding Cpk Formula
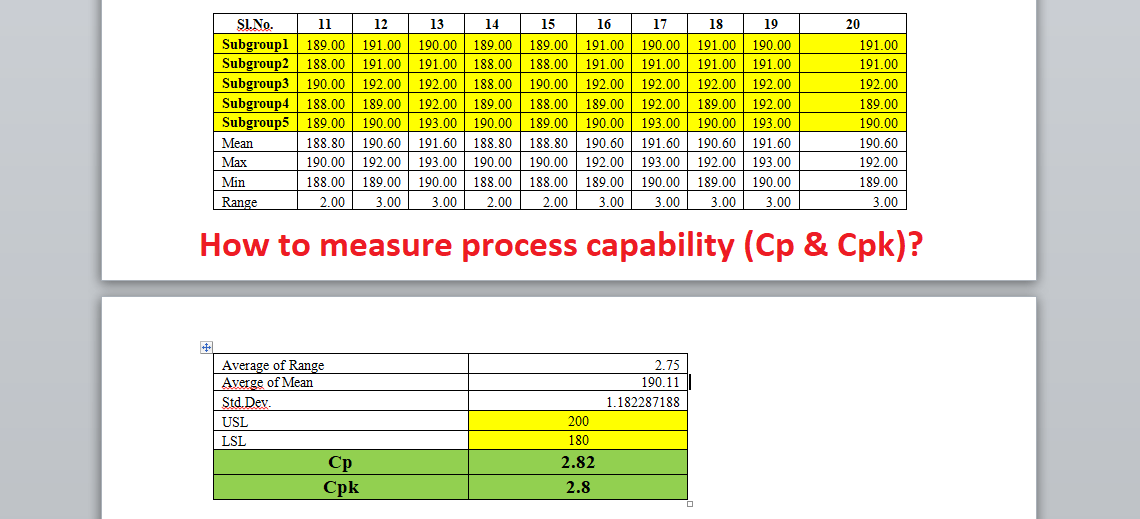
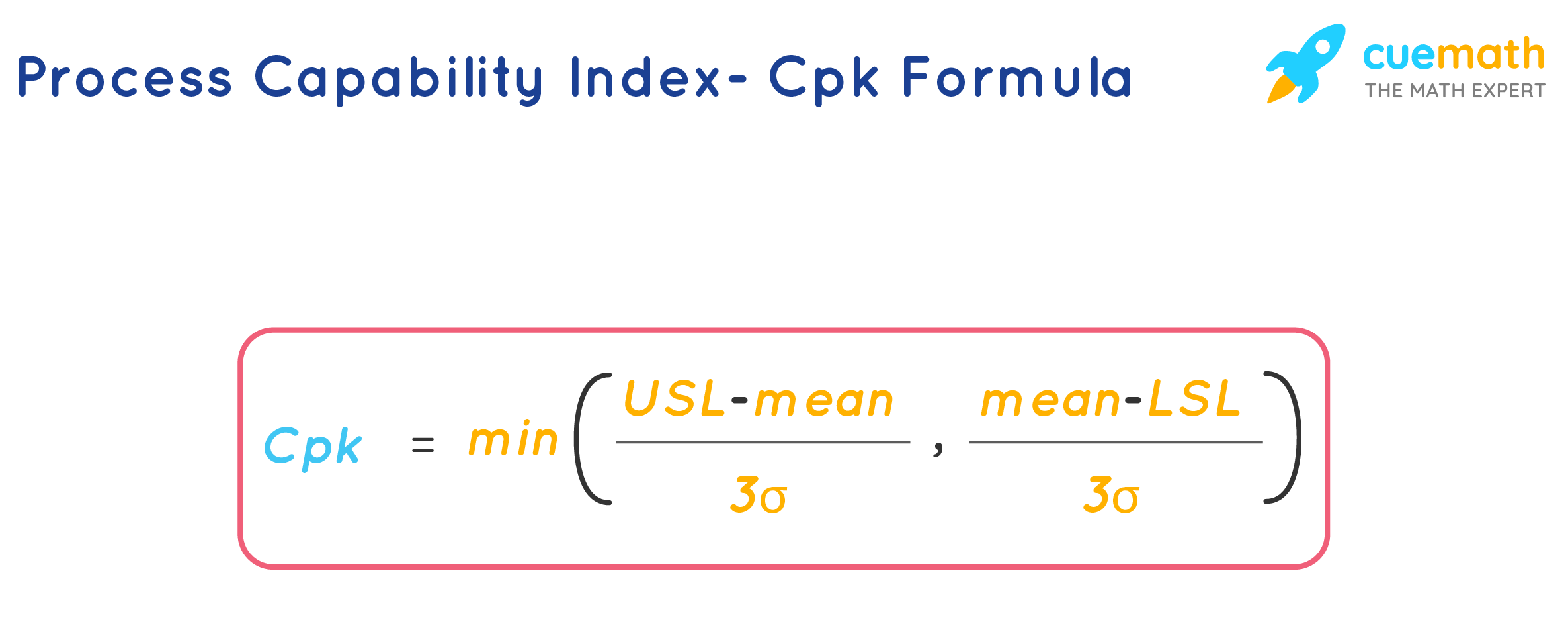
The Cpk formula is given by: Cpk = min(Cpk lower, Cpk upper) where, - Cpk lower = (Mean - Lower Specification Limit) / (3 * Standard Deviation) - Cpk upper = (Upper Specification Limit - Mean) / (3 * Standard Deviation) To calculate Cpk in Excel, we first need to understand these components and how to calculate them.
Calculating Mean in Excel
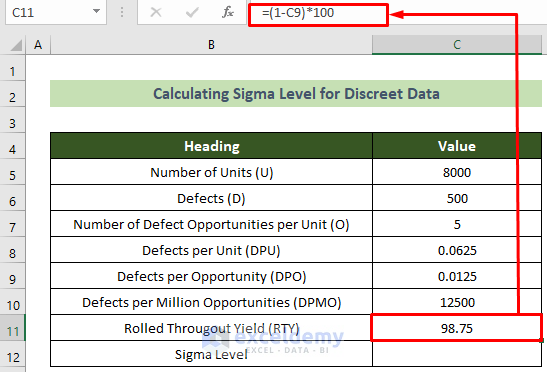
The mean of a dataset is calculated using the formula: Mean = (Σx) / n where Σx is the sum of all data points, and n is the number of data points. In Excel, we can calculate the mean using the =AVERAGE(range) function, where range is the range of cells containing the data.
Calculating Standard Deviation in Excel

The standard deviation of a dataset measures the amount of variation or dispersion from the mean. In Excel, we can calculate the standard deviation using the =STDEV.S(range) function for sample standard deviation or =STDEV.P(range) for population standard deviation.
Calculating Cpk Lower and Cpk Upper in Excel
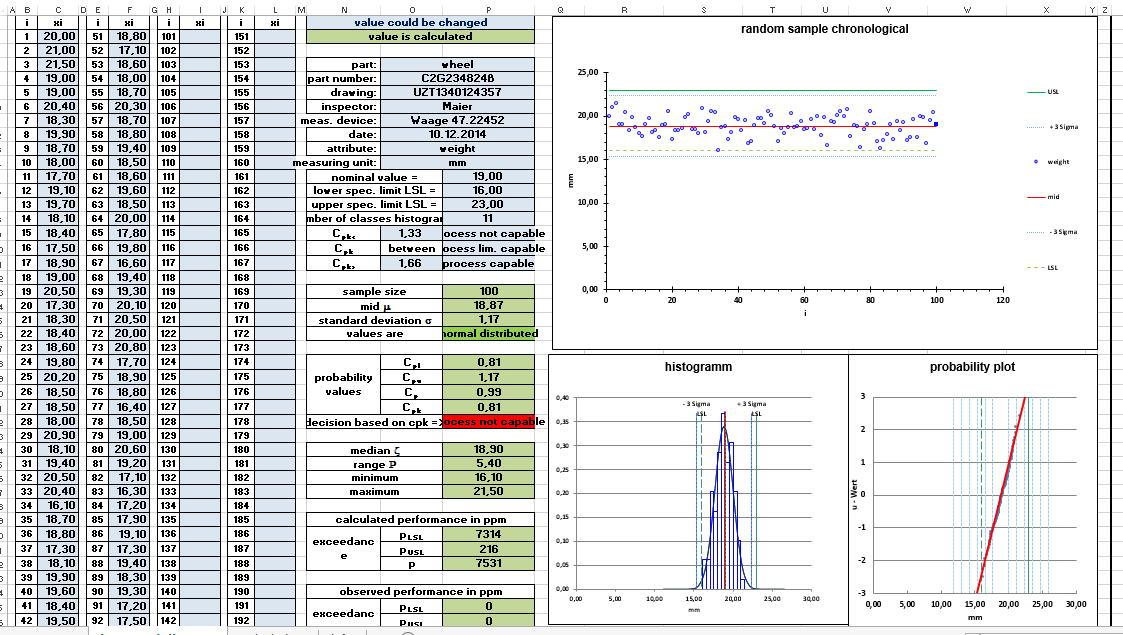
Using the mean and standard deviation calculated in the previous steps, we can now calculate Cpk lower and Cpk upper using the formulas: - Cpk lower = (Mean - Lower Specification Limit) / (3 * Standard Deviation) - Cpk upper = (Upper Specification Limit - Mean) / (3 * Standard Deviation) In Excel, we can use the following formulas, assuming the mean is in cell A1, the standard deviation is in cell A2, the lower specification limit is in cell A3, and the upper specification limit is in cell A4: - =((A1-A3)/(3*A2)) for Cpk lower - =((A4-A1)/(3*A2)) for Cpk upper
Calculating Final Cpk Value
The final Cpk value is the minimum of Cpk lower and Cpk upper. In Excel, we can use the =MIN(Cpk lower, Cpk upper) function, where Cpk lower and Cpk upper are the cells containing the calculated values.
Example Calculation
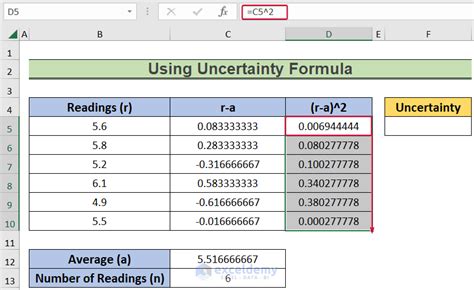
Let’s say we have a dataset with the following values: 10, 12, 11, 13, 12, and the lower specification limit is 9, and the upper specification limit is 15. First, calculate the mean: =AVERAGE(10, 12, 11, 13, 12) = 11.6 Then, calculate the standard deviation: =STDEV.S(10, 12, 11, 13, 12) = 1.14 Next, calculate Cpk lower and Cpk upper: - Cpk lower = ((11.6-9)/(3*1.14)) = 0.88 - Cpk upper = ((15-11.6)/(3*1.14)) = 1.13 Finally, calculate the Cpk value: =MIN(0.88, 1.13) = 0.88
| Specification | Value |
|---|---|
| Lower Specification Limit | 9 |
| Upper Specification Limit | 15 |
| Mean | 11.6 |
| Standard Deviation | 1.14 |
| Cpk Lower | 0.88 |
| Cpk Upper | 1.13 |
| Cpk Value | 0.88 |

💡 Note: The Cpk value indicates the capability of the process. A Cpk value greater than 1 indicates that the process is capable, while a value less than 1 indicates that the process is not capable.
To summarize, the key steps to calculate Cpk in Excel are: calculating the mean, calculating the standard deviation, calculating Cpk lower and Cpk upper, and finally, calculating the minimum of these two values to get the Cpk value. By following these steps, one can easily calculate the Cpk in Excel and understand the capability of a process.
What does Cpk measure?
+
Cpk measures the capability of a process to produce output within specified limits, comparing the width of a distribution to the specification limits.
How is Cpk calculated in Excel?

+
Cpk is calculated in Excel by first calculating the mean and standard deviation of the dataset, then using these values to calculate Cpk lower and Cpk upper, and finally taking the minimum of these two values.
What does a high Cpk value indicate?

+
A high Cpk value indicates that the process is more capable of producing output within the desired specifications.